State Round Materialmath Problem Solving
This section of the competition consists of 10 problems which the team has 20 minutes to complete. Team members may work together in any way to solve the problems. Team members may talk to each other during this section of the competition. This round assumes the use of calculators.
2020-21 Round 3 Solutions are now available.
Click here to register for the 2020-2021 USAMTS.
Stay informed and join our mailing list.

The USA Mathematical Talent Search (USAMTS) is a free mathematics competition open to all United States middle and high school students.
- Select a problem set using the buttons above, then use your mouse or tab key to select a question. Fill in the blank with the correct answer for each problem. When you have answered all of the questions, ask Charlie how you did. Round each number as indicated. Use commas correctly. Round 35 to the nearest ten. Round 243 to the nearest ten.
- As a result, it became evident that subject of problem solving has a positive effect on the development of mathematics teachers’ problem solving skills. Keywords: Mathematics Teaching, Problem Solving, Problem Solving Stages. Introduction Important mathematics concepts and procedures can be best taught through problem solving (Van De Walle.
Math Problem Solving
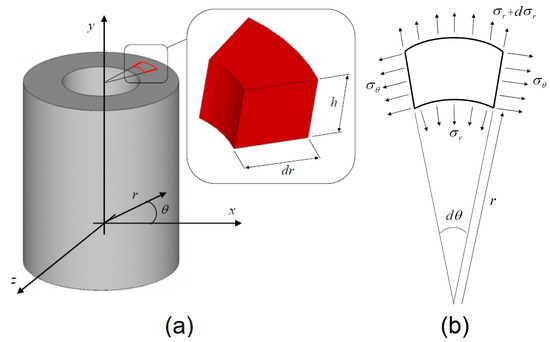
As opposed to most mathematics competitions, the USAMTS allows students a full month, or more, to work out their solutions. Carefully written justifications are required for each problem. The problems range in difficulty from being within the reach of most high school students to challenging the best students in the nation. Students may use any materials - books, calculators, computers - but all the work must be their own. The USAMTS is run on the honor system - it is an individual competition, whose competitive role is very secondary. (Although we do give prizes.)
download free, software usage tracker download free. Student solutions to the USAMTS problems are graded by mathematicians and comments are returned to the students. Our goal is to help all students develop their problem solving skills, improve their technical writing abilities, and mature mathematically while having fun. We wish to foster not only insight, ingenuity and creativity, but also the virtue of perseverance, which is equally essential in scientific endeavors.
Click on one of the links on the left side of the page to learn more about the USAMTS, view current or past problems, or to register (for free!) for the contest. Also, please join the mailing list to stay informed about the USAMTS.
Donations to help support the USAMTS are greatly appreciated.
The USAMTS is primarily sponsored by the National Security Agency and other sponsors.
As we discussed at the beginning of this section, hyperbolas have real-world applications in many fields, such as astronomy, physics, engineering, and architecture. The design efficiency of hyperbolic cooling towers is particularly interesting. Cooling towers are used to transfer waste heat to the atmosphere and are often touted for their ability to generate power efficiently. Because of their hyperbolic form, these structures are able to withstand extreme winds while requiring less material than any other forms of their size and strength. For example, a 500-foot tower can be made of a reinforced concrete shell only 6 or 8 inches wide!
Figure 10. Cooling towers at the Drax power station in North Yorkshire, United Kingdom (credit: Les Haines, Flickr)
The first hyperbolic towers were designed in 1914 and were 35 meters high. Today, the tallest cooling towers are in France, standing a remarkable 170 meters tall. In Example 6 we will use the design layout of a cooling tower to find a hyperbolic equation that models its sides.
Download eclipse ajax tools framework free. Download AJAX Toolkit Framework for free. AJAX Toolkit Framework is a J2EE based technology that assists in constructing Eclipse framework support for AJAX toolkits on Linux and provides enhanced DHTML/Javascript IDE features for AJAX developers. The Eclipse Ajax Tools Framework (ATF) is a tool integrated with Eclipse for Web developers who use Ajax techniques. ATF provides tooling that allows a user to edit, debug, and monitor CSS, HTML, and JavaScript applications and a framework on which adopters can build advanced and technology specific tools.

Example 6: Solving Applied Problems Involving Hyperbolas
The design layout of a cooling tower is shown in Figure 11. The tower stands 179.6 meters tall. The diameter of the top is 72 meters. At their closest, the sides of the tower are 60 meters apart.
Figure 11. Project design for a natural draft cooling tower
Find the equation of the hyperbola that models the sides of the cooling tower. Assume that the center of the hyperbola—indicated by the intersection of dashed perpendicular lines in the figure—is the origin of the coordinate plane. Round final values to four decimal places.
Solution
We are assuming the center of the tower is at the origin, so we can use the standard form of a horizontal hyperbola centered at the origin: [latex]frac{{x}^{2}}{{a}^{2}}-frac{{y}^{2}}{{b}^{2}}=1[/latex], where the branches of the hyperbola form the sides of the cooling tower. We must find the values of [latex]{a}^{2}[/latex] and [latex]{b}^{2}[/latex] to complete the model.
First, we find [latex]{a}^{2}[/latex]. Recall that the length of the transverse axis of a hyperbola is [latex]2a[/latex]. This length is represented by the distance where the sides are closest, which is given as [latex]text{ }65.3text{ }[/latex] meters. So, [latex]2a=60[/latex]. Therefore, [latex]a=30[/latex] and [latex]{a}^{2}=900[/latex].
To solve for [latex]{b}^{2}[/latex], we need to substitute for [latex]x[/latex] and [latex]y[/latex] in our equation using a known point. To do this, we can use the dimensions of the tower to find some point [latex]left(x,yright)[/latex] that lies on the hyperbola. We will use the top right corner of the tower to represent that point. Since the y-axis bisects the tower, our x-value can be represented by the radius of the top, or 36 meters. The y-value is represented by the distance from the origin to the top, which is given as 79.6 meters. Therefore,

The sides of the tower can be modeled by the hyperbolic equation
Math Solving Calculator
Try It 6
Solving Math Word Problems Free
A design for a cooling tower project is shown in Figure 12. Find the equation of the hyperbola that models the sides of the cooling tower. Assume that the center of the hyperbola—indicated by the intersection of dashed perpendicular lines in the figure—is the origin of the coordinate plane. Round final values to four decimal places.
